A Triangle Cannot Have More Than One Obtuse Angle

By Bio_Destiney85 11 May, 2022 Post a Comment

Now let us inspect triangle ABC. A line segment is a fixed portion of a line.

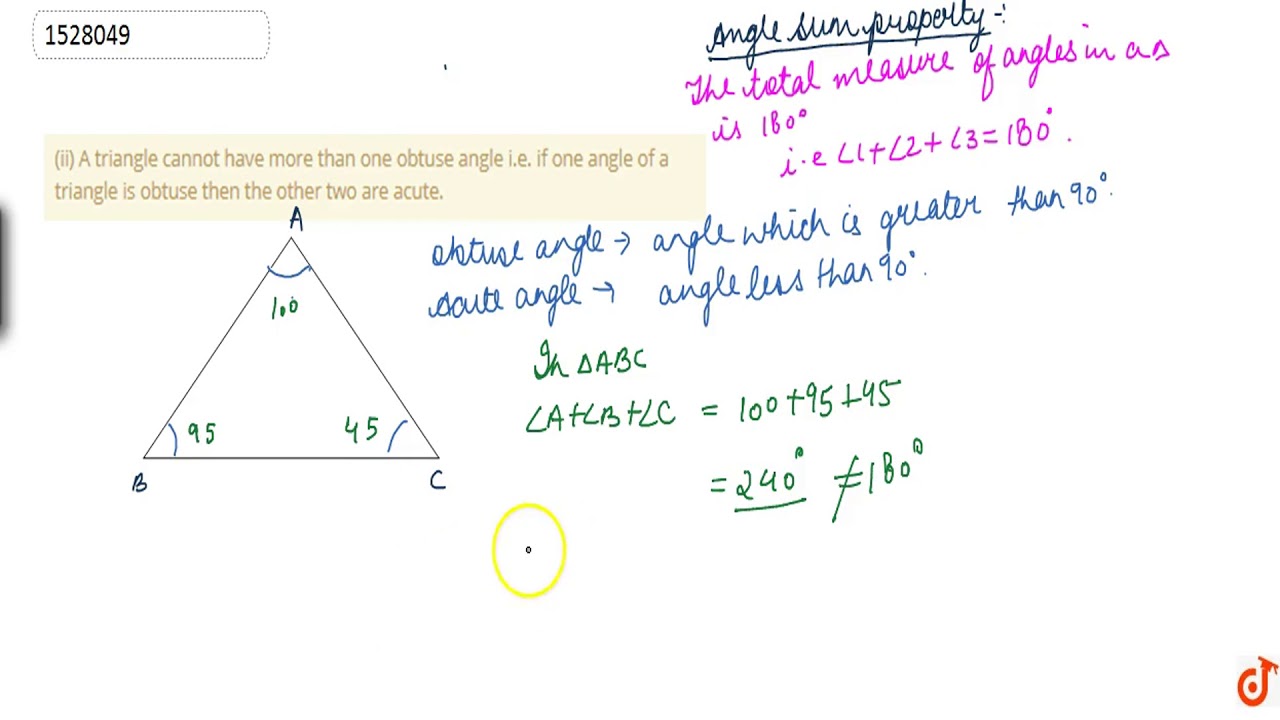
Ii A Triangle Cannot Have More Than One Obtuse Angle Youtube

One leg can be longer than the hypotenuse.

. You cannot add. As angles in a triangle add up to 180o the angle at C will be 180 degrees minus the sum of the other two angles. Triangles and the number three.

An obtuse triangle has any of its one angles more than 90. To deliver on this promise the mathematics standards are designed to address the problem of a. Even in a non-simple polygon it may be possible to define the exterior angle but one will have to pick an orientation of the plane or surface to decide the sign of the exterior angle measure.

A triangle with all its angles measuring less than 90 is called an acute triangle whereas a triangle with one angle measuring more than 90 is called an obtuse triangle. Triangles come in different sizes and dimensions however there are some properties that all triangles have in common. The wind chill formula from Exercise 1214 is only valid if the wind speed is above 3MPH and below 110MPH and the temperature is below 50 degrees Fahrenheit and above -50 degrees.

An acute triangle has all of its angles less than 90. Yes it is correct because the two angles make up a 90 angle. 54 billion people Lesson 131 1-68.

Since A is 120 degrees the sum of B and C will be less than 90 degrees. BC and AC are radii and so we have another isosceles triangle. Acute angle an angle that is less than 90 addition a mathematical operation in which the sum of two numbers or quantities is calculated.

Each leg is shorter than the hypotenuse. Possible but diagram is very inaccurate e. In the above triangle A B C 180 because of the Angle Sum Property Since A 120.

An angle less than 90 degrees like the angles of a triangle. SAS Similarity Theorem Two triangles are similar if an angle of one triangle is congruent to an angle of another triangle and the corresponding sides including those angles are in proportion. The hypotenuse is always the longest side in a right triangle.

As we cannot say that the angles at A and B are the same as angle x in triangle ACD we say that both the. Can be rejected using Triangle Inequality or Pythagorean Theorem. 23 67 90 1-63.

Here θ is an obtuse angle between 90 and 180. Its measure is greater than right angle and less than straight angle. What do all Triangles have in Common.

This can be done in several ways. Here the triangle ABC is an obtuse triangle as A measures more than 90 degrees. Here AB BC CA.

What is a Right-Angle Triangle. A perimeter of a triangle is defined as the total length of the outer boundary of the. Third angle must be 59º by Triangle Angle Sum but side opposite 62º must be larger than side opposite 59º by Longest Side Largest Angle.

In a triangle the sum of the measures of the interior angles is 180º. The five types of angles are. For example all triangles have three sides and three angles the sum of the interior angles is.

A triangle which has one angle of 90. A triangle that has one of the interior angles as 90 degrees is a right-angled triangle. These kinds of more irregularly shaped triangles are less commonly use as symbols since they dont have the same power as equilateral triangles or isosceles triangles.

Triangle Angle-Bisector Theorem If a segment bisects an angle of a triangle then it divides the opposite side into segments proportional to the other two sides. In a right triangle one of the angles is equal to 90 or right angle. The sine of an obtuse angle is defined to be the sine of its supplementary.

Sensitive physical or intellectual perception like an acute sense of smell or an acute thinker. A triangle cannot have more than one obtuse angle. Frequently Asked Questions on Acute Angle FAQs.

The sum of the angles is 179. What are the 5 types of angles. ABC one right angle.

9465 people per square mile b. No triangle can have two obtuse angles. In an obtuse triangle if one angle measures more than 90 then the sum of the remaining two angles is less than 90.

A right triangle can have an obtuse angle. In Euclidean geometry the sum of the exterior angles of a simple. An equilateral triangle must have three angles that measure each.

When all side lengths of a triangle are equal. Both angles of a right triangle that are not right must be acute. The reference angle alpha α is equal to 180 θ and is the acute angle within the right-angled triangle.

A triangle is made of three a quadrilateral of four line segments. An angle larger than a right angle and smaller than a straight angle between 90 and 180 is called an obtuse angle. Acute angles 90 Right angles 90 Obtuse angles.

So when working in a triangle with sin A must be less that 1 and greater than -1. To compare any two line segments we find a relation between their lengths. A triangle with at least two sides of equal length is Isosceles triangle.

This is expressed as 180-2x. Its measure is less than a right angle. Characterized by sharpness or severity such as acute pain.

If the Reynolds number is less than 2000 print laminar flow if its between 2000 and 4000 print transient flow and if its more than 4000 print turbulent flow. In obtuse triangle any one of the triangles is greater than 90. For more than a decade research studies of mathematics education in high-performing countries have concluded that mathematics education in the United States must become substantially more focused and coherent in order to improve mathematics achievement in this country.

The measures of the angles of a right triangle can total. We use this idea to compare line segments. This measure of each line segment is a unique number called its length.

Here AB AC. In Diagram ii we have rotated the radius further in an anti-clockwise direction past the vertical y axis into the next quadrant. This makes it possible to measure a line segment.

A triangle can have more than one acute angle. Usually indicated by the symbol algorithm or algorism a recursive procedure whereby an infinite sequence of terms can be generated angle the extent to which one such line or plane diverges from another measured in. X33 so one angle is 33 10 23 while the other is 233 1 67.

4th Grade Math 10 2 Classify Triangles By Angles Youtube